ALGEBRA AND GEOMETRY WITH CALCULS

One of the earliest algebra topics learned is how to find the slope of a line--a numerical value that describes just how slanted that line is. Calculus gives us a much more generalized method of finding slopes. With it, we can find not only how steeply a line slopes, but indeed, how steeply any curve slopes at any given point.

Without calculus, it is difficult to find areas of shapes other than those whose formulas you learned in geometry. You may be able to find the area of commons shapes such as a triangle, square, rectangle, circle, and even a trapezoid; but how could you find the area of the shape like the one shown below?

With calculus, you can calculate complicated x-intercepts. Without a graphing calculator, it is pretty difficult to calculate an irrational root. However, a simple process called Newton's Method (named Isaac Newton) allows you to calculate an irrational root to whatever accuracy you want.

Calculus makes it much easier to visualize graphs. You may already have a good grasp of linear functions and how to visualize their graphs easily, but what about the graph of something like y= x^3 + 2x^2 - x + 1? Elementary calculus tells you exactly where that graph will be increasing, decreasing, and twisting. You can even find the highest and lowest points on the graph without plotting a single point.

One of the most useful applications of calculus is the optimization of functions. In a small number of steps, you can answer questions such as:

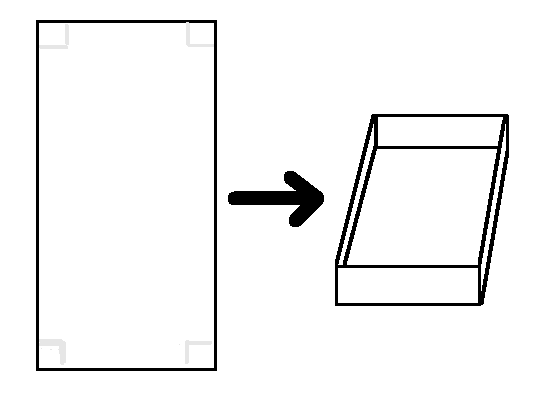
If I have 500 feet of fence, what is the largest rectangular yard I can make? or Given a rectangular sheet of paper which measures 8.5 inches by 11 inches, what are the dimensions of the box I can make containing the greatest volume?

The traditional way to create an open box from a rectangular surface is to cut congruent squares from the corners of the rectangle and then to fold the resulting sides up as shown:

Calculus develops concepts in other mathematics that lets us discover more about them and enables us to achieve greater feats than the mathematics that it is built on. It is vital to understanding and making sense of the world we live in.